Authors:
(1) Toshiki Kawai, Department of Physics, Hokkaido University, Sapporo 060-0810, Japan (E-mail: t-kawai@higgs3.sci.hokudai.ac.jp);
(2) Yoshiharu Kawamura, Department of Physics, Shinshu University, Matsumoto 390-8621, Japan (E-mail: haru@azusa.shinshu-u.ac.jp).
Table of Links
2 U(1) gauge theory on a warped background
3 Gauge-Higgs inflation on a warped background
4 Conclusions and discussions, Acknowledgements, and References
2 U(1) gauge theory on a warped background
2.1 Randall-Sundrum metric and action integral
The spacetime is assumed to be 5d one with the RS metric given by [8, 9]
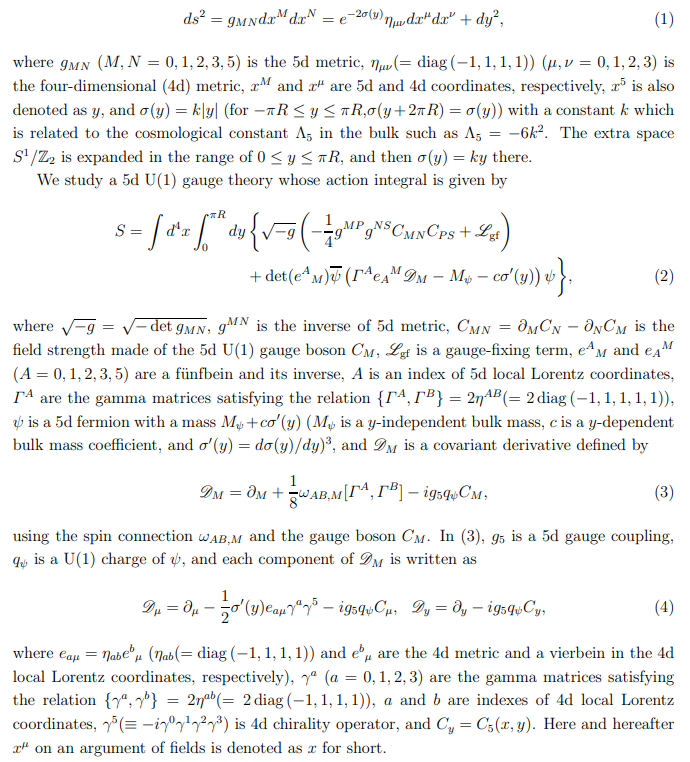
2.2 Conjugate boundary conditions
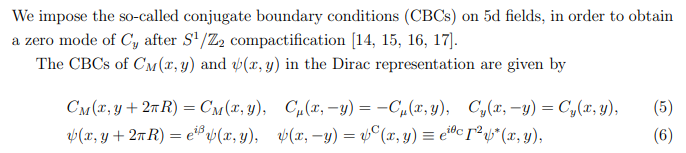
where β is a constant called a twisted phase, the superscript C denotes a 4d charge conjugation, θC is a real number, and the asterisk means the complex conjugation. Then, the covariant derivatives obey the relations:
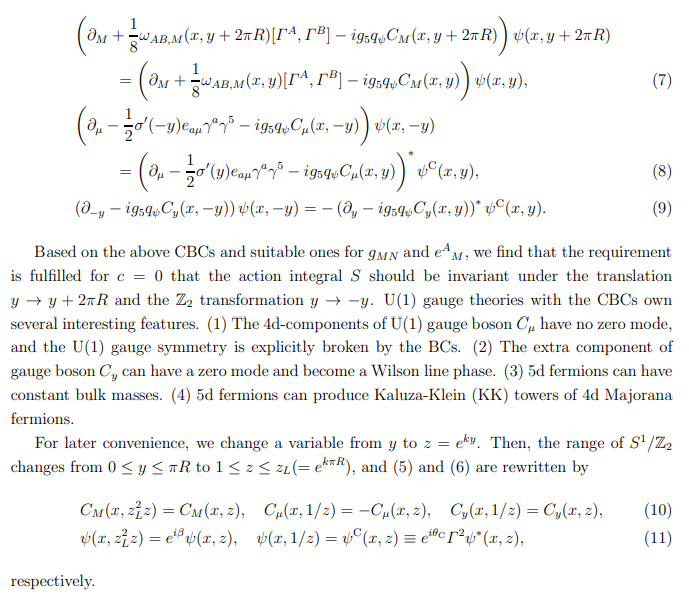
2.3 Mass spectrum
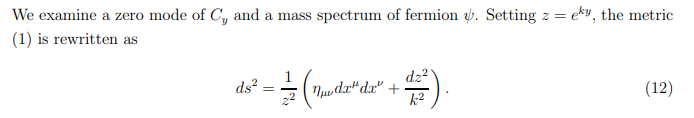
Then, the action integral is rewritten as


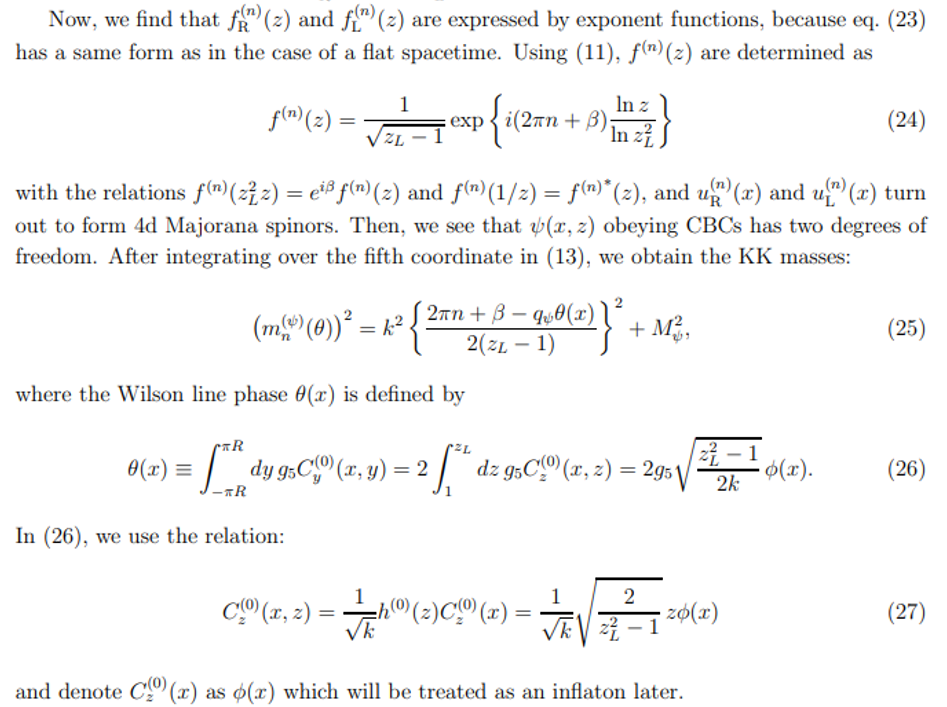
2.4 Effective potential
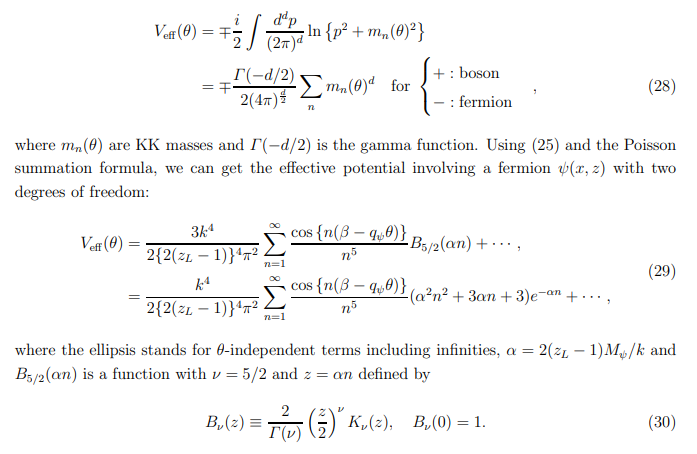
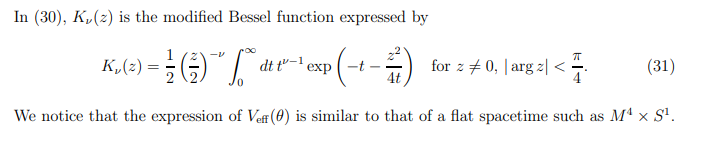
Let us derive the effective potential for the Wilson line phase θ(= θ(x)). Taking the standard procedure, a d-dimensional effective potential involving one degree of freedom at the one-loop level is given b
This paper is available on arxiv under CC BY 4.0 DEED license.
[3] We introduce both Mψ and cσ′ (y) in a general standpoint, and we will see that cσ′ (y) is forbidden by imposing specific boundary conditions on fields in the next subsection.
